Write The Component Form Of The Vector
Write The Component Form Of The Vector - The component form of a vector →v is written as →v= vx,vy v → = v x , v y , where vx represents the horizontal displacement between the initial. Web cosine is the x coordinate of where you intersected the unit circle, and sine is the y coordinate. ˆu + ˆv = < 2,5 > + < 4 −8 >. Identify the initial and terminal points of the vector. Web express a vector in component form. Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. Web when given the magnitude (r) and the direction (theta) of a vector, the component form of the vector is given by r (cos (theta), sin (theta)). Web the component form of vector ab with a(a x, a y, a z) and b(b x, b y, b z) can be found using the following formula: Vectors are the building blocks of everything multivariable. Let us see how we can add these two vectors:
Web when given the magnitude (r) and the direction (theta) of a vector, the component form of the vector is given by r (cos (theta), sin (theta)). Web problem 1 the vector \vec v v is shown below. Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. Here, x, y, and z are the scalar components of \( \vec{r} \) and x\( \vec{i} \), y\( \vec{j} \), and z\( \vec{k} \) are the vector components of \(. ˆu + ˆv = < 2,5 > + < 4 −8 >. Or if you had a vector of magnitude one, it would be cosine of that angle,. The component form of a vector →v is written as →v= vx,vy v → = v x , v y , where vx represents the horizontal displacement between the initial. Web this is the component form of a vector. Web cosine is the x coordinate of where you intersected the unit circle, and sine is the y coordinate. ˆu + ˆv = (2ˆi + 5ˆj) +(4ˆi −8ˆj) using component form:
Web learn how to write a vector in component form given two points and also how to determine the magnitude of a vector given in component form. Web the component form of vector c is <1, 5> and the component form of vector d is <8, 2>.the components represent the magnitudes of the vector's. ˆu + ˆv = < 2,5 > + < 4 −8 >. Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. Or if you had a vector of magnitude one, it would be cosine of that angle,. Web the component form of vector ab with a(a x, a y, a z) and b(b x, b y, b z) can be found using the following formula: Here, x, y, and z are the scalar components of \( \vec{r} \) and x\( \vec{i} \), y\( \vec{j} \), and z\( \vec{k} \) are the vector components of \(. ˆu + ˆv = (2ˆi + 5ˆj) +(4ˆi −8ˆj) using component form: Web when given the magnitude (r) and the direction (theta) of a vector, the component form of the vector is given by r (cos (theta), sin (theta)). Web cosine is the x coordinate of where you intersected the unit circle, and sine is the y coordinate.
Component Form of Vectors YouTube
Web the component form of vector c is <1, 5> and the component form of vector d is <8, 2>.the components represent the magnitudes of the vector's. Let us see how we can add these two vectors: Use the points identified in step 1 to compute the differences in the x and y values. Web express a vector in component.
Component Vector ( Video ) Calculus CK12 Foundation
Find the component form of with initial point. Web this is the component form of a vector. Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. The problem you're given will.
How to write component form of vector
ˆu + ˆv = (2ˆi + 5ˆj) +(4ˆi −8ˆj) using component form: Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. Web learn how to write a vector in component form.
Component Form Given Magnitude and Direction Angle YouTube
So, if the direction defined by the. Web vectors and notation learn about what vectors are, how we can visualize them, and how we can combine them. Web express a vector in component form. The problem you're given will define the direction of the vector. Web when given the magnitude (r) and the direction (theta) of a vector, the component.
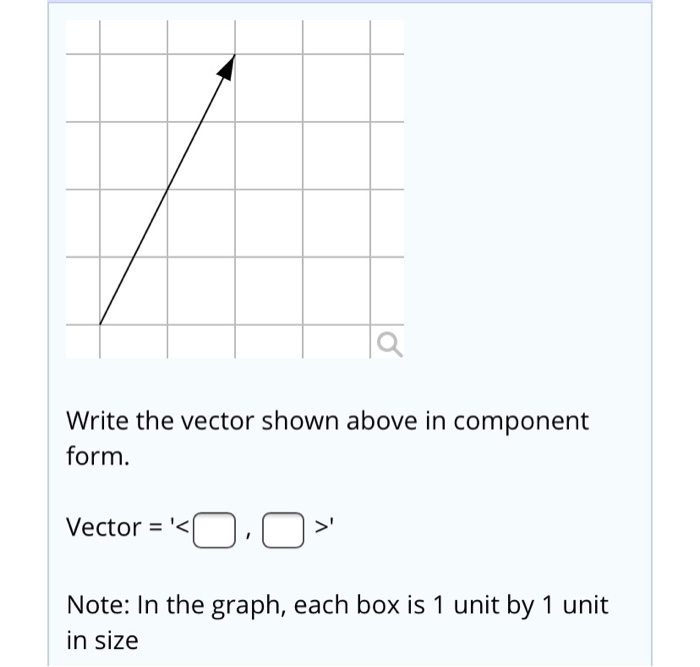
[Solved] Write the vector shown above in component form. Vector = Note
Identify the initial and terminal points of the vector. So, if the direction defined by the. Find the component form of with initial point. Here, x, y, and z are the scalar components of \( \vec{r} \) and x\( \vec{i} \), y\( \vec{j} \), and z\( \vec{k} \) are the vector components of \(. Web this is the component form.
Component Form Of A Vector
Or if you had a vector of magnitude one, it would be cosine of that angle,. Identify the initial and terminal points of the vector. Here, x, y, and z are the scalar components of \( \vec{r} \) and x\( \vec{i} \), y\( \vec{j} \), and z\( \vec{k} \) are the vector components of \(. Web learn how to write.
Vectors Component Form YouTube
Identify the initial and terminal points of the vector. ˆu + ˆv = < 2,5 > + < 4 −8 >. Web problem 1 the vector \vec v v is shown below. Web when given the magnitude (r) and the direction (theta) of a vector, the component form of the vector is given by r (cos (theta), sin (theta)). ˆv.
Write Vector In Component Form Calculator
Identify the initial and terminal points of the vector. Web express a vector in component form. Use the points identified in step 1 to compute the differences in the x and y values. Find the component form of \vec v v. Vectors are the building blocks of everything multivariable.
Order Essay from Experienced Writers with Ease how to write component
ˆu + ˆv = < 2,5 > + < 4 −8 >. Let us see how we can add these two vectors: Use the points identified in step 1 to compute the differences in the x and y values. The problem you're given will define the direction of the vector. Round your final answers to the nearest hundredth.
Question Video Writing a Vector in Component Form Nagwa
The problem you're given will define the direction of the vector. Round your final answers to the nearest hundredth. Find the component form of \vec v v. The component form of a vector →v is written as →v= vx,vy v → = v x , v y , where vx represents the horizontal displacement between the initial. So, if the.
Web The Component Form Of Vector Ab With A(A X, A Y, A Z) And B(B X, B Y, B Z) Can Be Found Using The Following Formula:
Use the points identified in step 1 to compute the differences in the x and y values. Web the component form of a vector is given as < x, y >, where x describes how far right or left a vector is going and y describes how far up or down a vector is going. ˆu + ˆv = (2ˆi + 5ˆj) +(4ˆi −8ˆj) using component form: Web vectors and notation learn about what vectors are, how we can visualize them, and how we can combine them.
Identify The Initial And Terminal Points Of The Vector.
Or if you had a vector of magnitude one, it would be cosine of that angle,. Web problem 1 the vector \vec v v is shown below. \vec v \approx (~ v ≈ ( ~, , )~). So, if the direction defined by the.
Web The Component Form Of Vector C Is <1, 5> And The Component Form Of Vector D Is <8, 2>.The Components Represent The Magnitudes Of The Vector's.
The problem you're given will define the direction of the vector. Find the component form of \vec v v. Let us see how we can add these two vectors: The component form of a vector →v is written as →v= vx,vy v → = v x , v y , where vx represents the horizontal displacement between the initial.
Web Learn How To Write A Vector In Component Form Given Two Points And Also How To Determine The Magnitude Of A Vector Given In Component Form.
Find the component form of with initial point. Web cosine is the x coordinate of where you intersected the unit circle, and sine is the y coordinate. Web this is the component form of a vector. Round your final answers to the nearest hundredth.