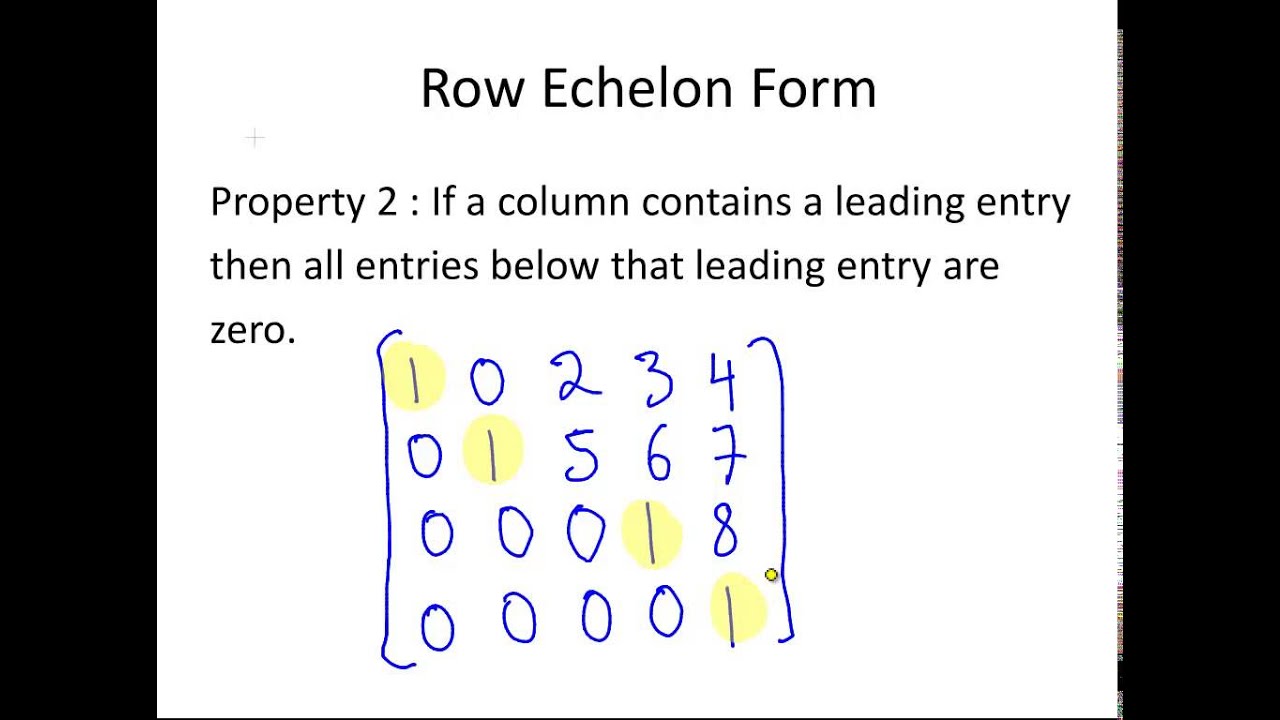
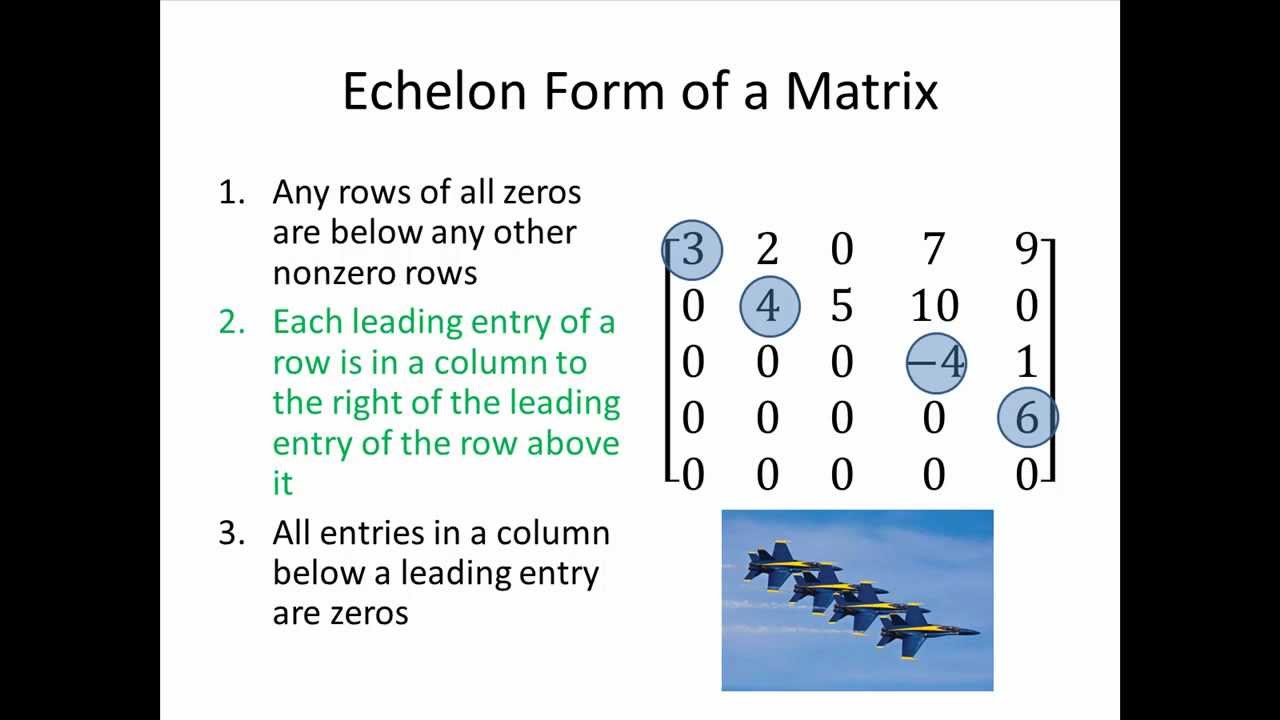
Is The Echelon Form Of A Matrix Unique
Is The Echelon Form Of A Matrix Unique - Can any two matrices of the same size be multiplied? If a matrix reduces to two reduced matrices r and s, then we need to show r = s. 6 claim that multiplication by these elementary matrices from the left amounts exactly to three. A matrix is said to be in. The echelon form of a matrix is unique. The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process. Algebra and number theory | linear algebra | systems of linear equations. Both the echelon form and the. Web so r 1 and r 2 in a matrix in echelon form becomes as follows: The leading entry in row 1 of matrix a is to the.
Web example (reduced echelon form) 2 6 6 6 6 4 0 1 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 1 3 7 7 7 7 5 theorem (uniqueness of the reduced echelon. Web here i start with the identity matrix and put at the i; We're talking about how a row echelon form is not unique. Web the reason that your answer is different is that sal did not actually finish putting the matrix in reduced row echelon form. If a matrix reduces to two reduced matrices r and s, then we need to show r = s. I am wondering how this can possibly be a unique matrix when any nonsingular matrix is row equivalent to. The other matrices fall short. For a matrix to be in rref every leading (nonzero). Web if the statement is false, then correct it and make it true. This leads us to introduce the next definition:
Algebra and number theory | linear algebra | systems of linear equations. So let's take a simple matrix that's. We're talking about how a row echelon form is not unique. For a matrix to be in rref every leading (nonzero). This leads us to introduce the next definition: A matrix is said to be in. 6 claim that multiplication by these elementary matrices from the left amounts exactly to three. Can any two matrices of the same size be multiplied? The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process. Web example (reduced echelon form) 2 6 6 6 6 4 0 1 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 1 0 0 0 0 0 0 0 0 0 1 3 7 7 7 7 5 theorem (uniqueness of the reduced echelon.
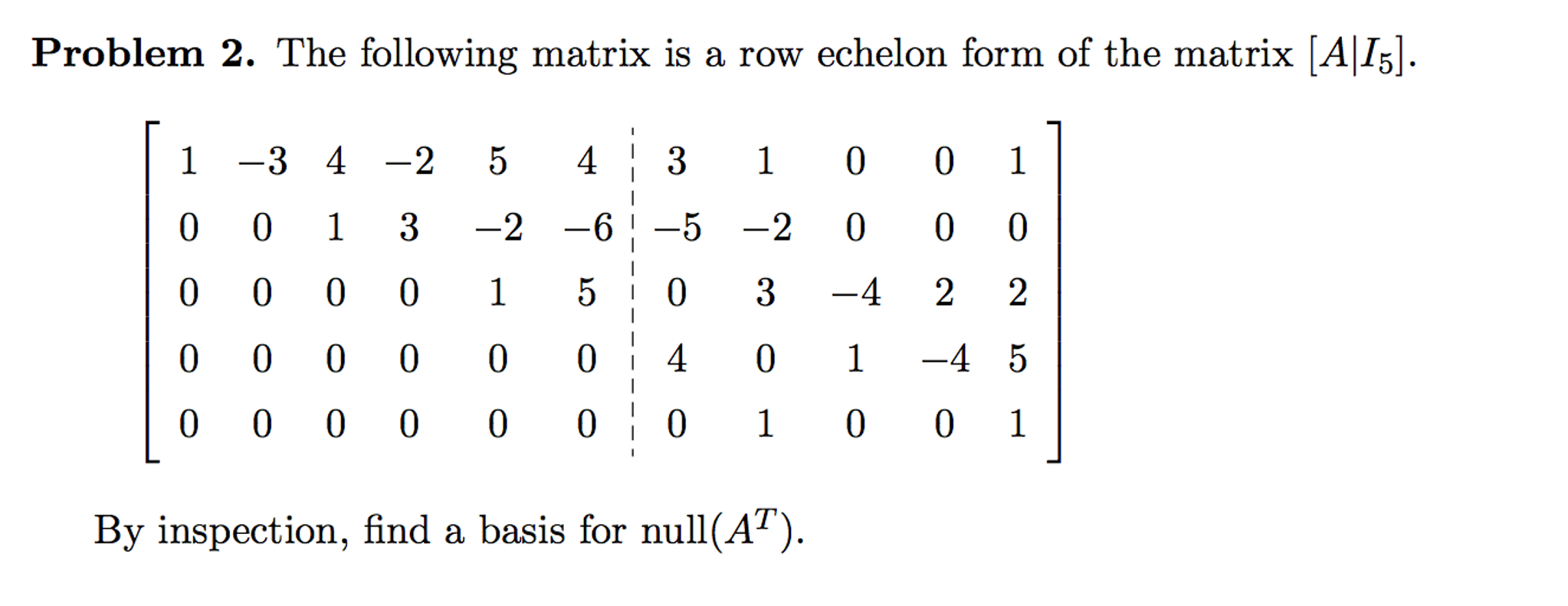
Solved The following matrix is a row echelon form of the
Web here i start with the identity matrix and put at the i; Web so r 1 and r 2 in a matrix in echelon form becomes as follows: 6 claim that multiplication by these elementary matrices from the left amounts exactly to three. The leading entry in row 1 of matrix a is to the. ☆ ☆☆☆☆ ☆☆☆☆ ☆☆☆☆.
Uniqueness of Reduced Row Echelon Form YouTube
Web so r 1 and r 2 in a matrix in echelon form becomes as follows: Here we will prove that. The answer to this question lies with properly understanding the reduced. Web algebra questions and answers. The other matrices fall short.
7.3.3 Row Echelon Form of a Matrix YouTube
The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process. Web one sees the solution is z = −1, y = 3, and x = 2. Web so r 1 and r 2 in a matrix in echelon form becomes as follows: Algebra and number theory | linear algebra | systems of.
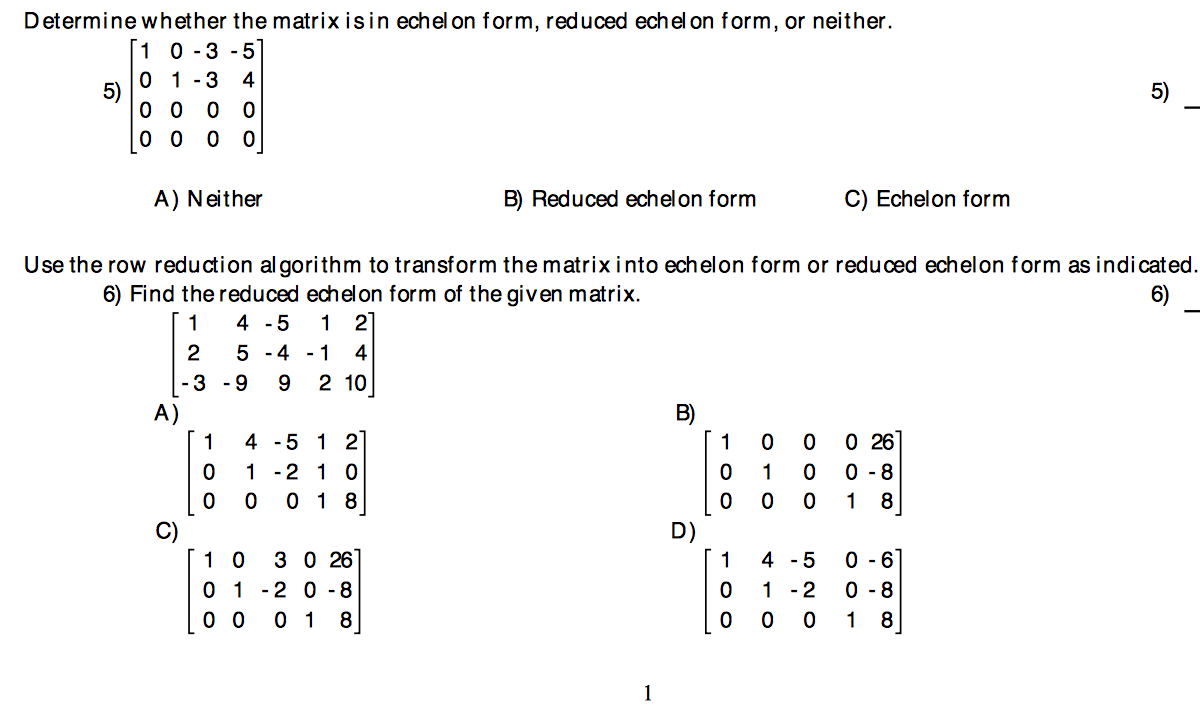
Solved Determine whether the matrix isin echelon form,
Both the echelon form and the. The echelon form of a matrix is unique. Web solution the correct answer is (b), since it satisfies all of the requirements for a row echelon matrix. If a matrix reduces to two reduced matrices r and s, then we need to show r = s. Web the reason that your answer is different.
Solved The reduced echelon form of a matrix is unique.
So there is a unique solution to the original system of equations. Web here i start with the identity matrix and put at the i; The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process. The echelon form of a matrix is unique. Here we will prove that.
Solved Suppose The Reduced Row Echelon Form Of The Matrix...
Web if the statement is false, then correct it and make it true. The other matrices fall short. Both the echelon form and the. Web so r 1 and r 2 in a matrix in echelon form becomes as follows: The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process.
Echlon Form How To Reduce A Matrix To Row Echelon Form 8 Steps
Both the echelon form and the. I am wondering how this can possibly be a unique matrix when any nonsingular matrix is row equivalent to. Web algebra questions and answers. This leads us to introduce the next definition: For a matrix to be in rref every leading (nonzero).
Row Echelon Form of a Matrix YouTube
If a matrix reduces to two reduced matrices r and s, then we need to show r = s. So there is a unique solution to the original system of equations. Web how can we tell what kind of solution (if one exists) a given system of linear equations has? The echelon form of a matrix is unique. The answer.
ROW ECHELON FORM OF A MATRIX. YouTube
The echelon form of a matrix is unique. 6 claim that multiplication by these elementary matrices from the left amounts exactly to three. So let's take a simple matrix that's. The reduced (row echelon) form of a matrix is unique. Choose the correct answer below.
Elementary Linear Algebra Echelon Form of a Matrix, Part 1 YouTube
Web the reason that your answer is different is that sal did not actually finish putting the matrix in reduced row echelon form. Web here i start with the identity matrix and put at the i; This leads us to introduce the next definition: The other matrices fall short. I am wondering how this can possibly be a unique matrix.
Web So R 1 And R 2 In A Matrix In Echelon Form Becomes As Follows:
Web the reason that your answer is different is that sal did not actually finish putting the matrix in reduced row echelon form. The echelon form of a matrix is unique. Both the echelon form and the. So there is a unique solution to the original system of equations.
If A Matrix Reduces To Two Reduced Matrices R And S, Then We Need To Show R = S.
Here we will prove that. Web if the statement is false, then correct it and make it true. Web how can we tell what kind of solution (if one exists) a given system of linear equations has? So let's take a simple matrix that's.
This Leads Us To Introduce The Next Definition:
The other matrices fall short. The leading entry in row 1 of matrix a is to the. Can any two matrices of the same size be multiplied? And the easiest way to explain why is just to show it with an example.
Web Here I Start With The Identity Matrix And Put At The I;
A matrix is said to be in. The echelon form of a matrix is unique. The pivot positions in a matrix depend on whether row interchanges are used in the row reduction process. Choose the correct answer below.