Flux Form Of Green's Theorem
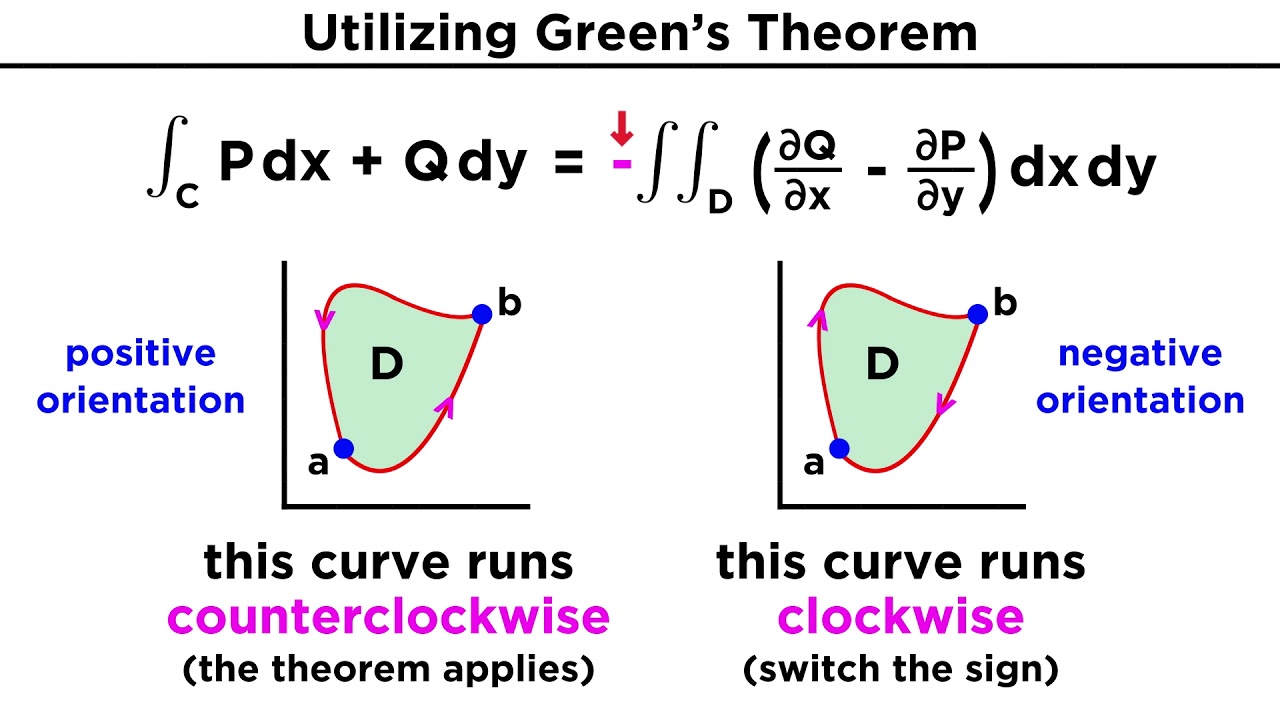
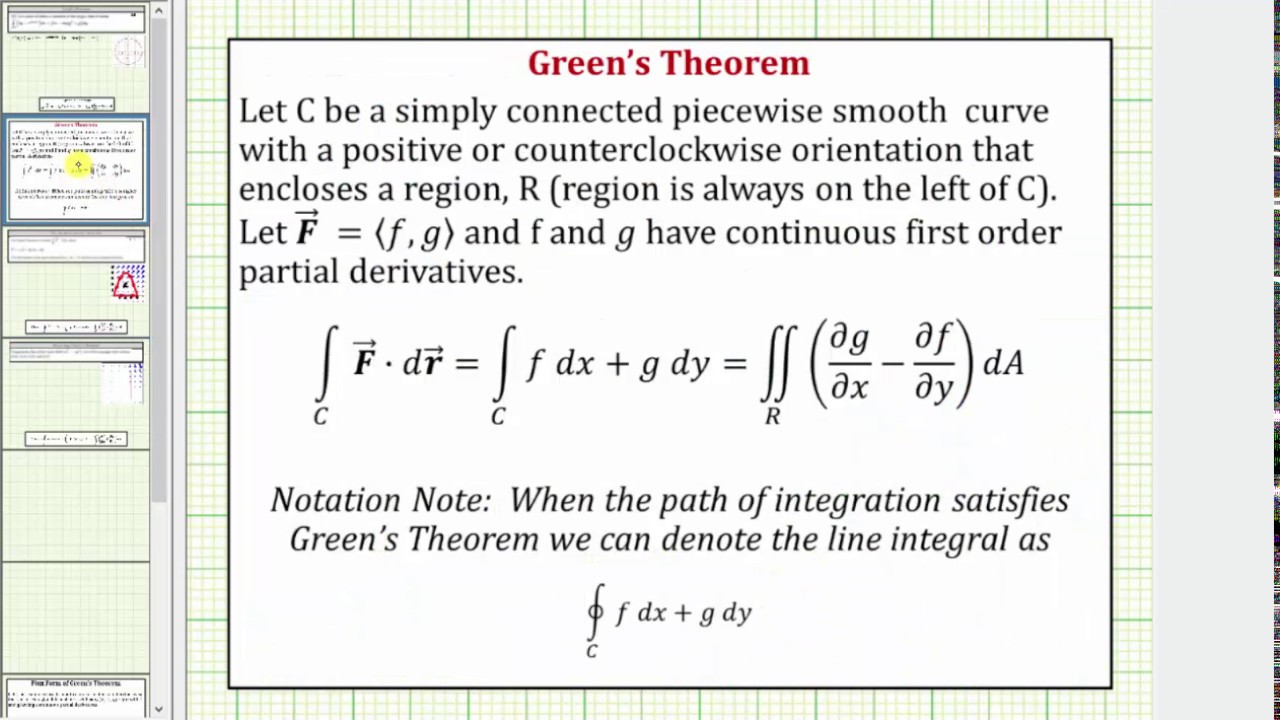
Flux Form Of Green's Theorem - A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Its the same convention we use for torque and measuring angles if that helps you remember All four of these have very similar intuitions. Web circulation form of green's theorem google classroom assume that c c is a positively oriented, piecewise smooth, simple, closed curve. Web green's theorem is a vector identity which is equivalent to the curl theorem in the plane. Start with the left side of green's theorem: Green's theorem allows us to convert the line integral into a double integral over the region enclosed by c. It relates the line integral of a vector field around a planecurve to a double integral of “the derivative” of the vector field in the interiorof the curve. Web it is my understanding that green's theorem for flux and divergence says ∫ c φf =∫ c pdy − qdx =∬ r ∇ ⋅f da ∫ c φ f → = ∫ c p d y − q d x = ∬ r ∇ ⋅ f → d a if f =[p q] f → = [ p q] (omitting other hypotheses of course). Using green's theorem in its circulation and flux forms, determine the flux and circulation of f around the triangle t, where t is the triangle with vertices ( 0, 0), ( 1, 0), and ( 0, 1), oriented counterclockwise.
Using green's theorem in its circulation and flux forms, determine the flux and circulation of f around the triangle t, where t is the triangle with vertices ( 0, 0), ( 1, 0), and ( 0, 1), oriented counterclockwise. All four of these have very similar intuitions. In the flux form, the integrand is f⋅n f ⋅ n. The line integral in question is the work done by the vector field. Web in this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. In this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. F ( x, y) = y 2 + e x, x 2 + e y. Web using green's theorem to find the flux. Green’s theorem has two forms: For our f f →, we have ∇ ⋅f = 0 ∇ ⋅ f → = 0.
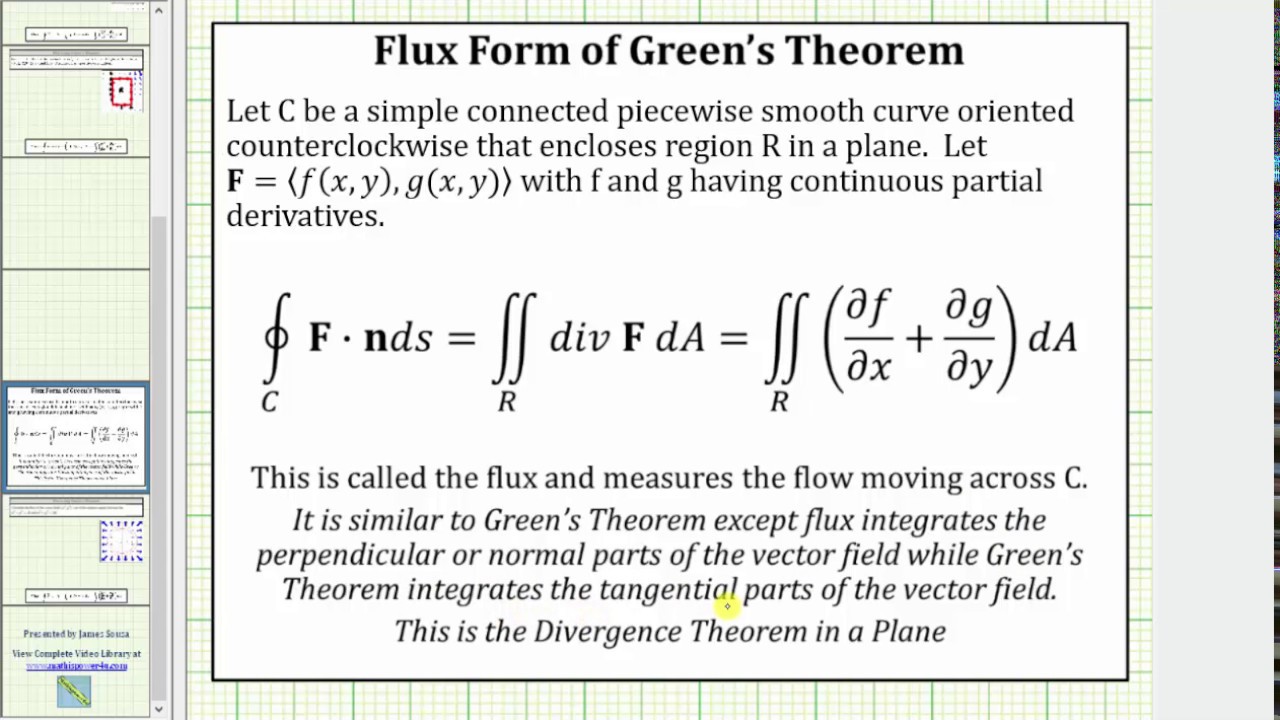
A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Green's theorem allows us to convert the line integral into a double integral over the region enclosed by c. An interpretation for curl f. The line integral in question is the work done by the vector field. In the flux form, the integrand is f⋅n f ⋅ n. Proof recall that ∮ f⋅nds = ∮c−qdx+p dy ∮ f ⋅ n d s = ∮ c − q d x + p d y. F ( x, y) = y 2 + e x, x 2 + e y. Then we will study the line integral for flux of a field across a curve. Web flux form of green's theorem. The flux of a fluid across a curve can be difficult to calculate using the flux line integral.
multivariable calculus How are the two forms of Green's theorem are
Using green's theorem in its circulation and flux forms, determine the flux and circulation of f around the triangle t, where t is the triangle with vertices ( 0, 0), ( 1, 0), and ( 0, 1), oriented counterclockwise. Web the flux form of green’s theorem relates a double integral over region \(d\) to the flux across boundary \(c\). A.
Calculus 3 Sec. 17.4 Part 2 Green's Theorem, Flux YouTube
Start with the left side of green's theorem: Web green’s theorem states that ∮ c f → ⋅ d r → = ∬ r curl f → d a; It relates the line integral of a vector field around a planecurve to a double integral of “the derivative” of the vector field in the interiorof the.
Green's Theorem YouTube
Web math multivariable calculus unit 5: An interpretation for curl f. In this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. All four of these have very similar intuitions. F ( x, y) = y 2 + e x, x 2 + e y.
Flux Form of Green's Theorem Vector Calculus YouTube
Green’s theorem has two forms: Note that r r is the region bounded by the curve c c. Web 11 years ago exactly. Web we explain both the circulation and flux forms of green's theorem, and we work two examples of each form, emphasizing that the theorem is a shortcut for line integrals when the curve is a boundary. The.
Determine the Flux of a 2D Vector Field Using Green's Theorem (Hole
Because this form of green’s theorem contains unit normal vector n n, it is sometimes referred to as the normal form of green’s theorem. This video explains how to determine the flux of a. Since curl f → = 0 , we can conclude that the circulation is 0 in two ways. Green’s theorem has two forms: Web 11.
Flux Form of Green's Theorem YouTube
Web first we will give green’s theorem in work form. Web in this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. Proof recall that ∮ f⋅nds = ∮c−qdx+p dy ∮ f ⋅ n d s = ∮ c − q d x + p d y. Web green's theorem is.
Determine the Flux of a 2D Vector Field Using Green's Theorem (Parabola
A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Green’s theorem has two forms: It relates the line integral of a vector field around a planecurve to a double integral of “the derivative” of the vector field in the interiorof the curve. Web we explain both the circulation.
Green's Theorem Flux Form YouTube
Since curl f → = 0 in this example, the double integral is simply 0 and hence the circulation is 0. Start with the left side of green's theorem: Tangential form normal form work by f flux of f source rate around c across c for r 3. It relates the line integral of a vector field around a.
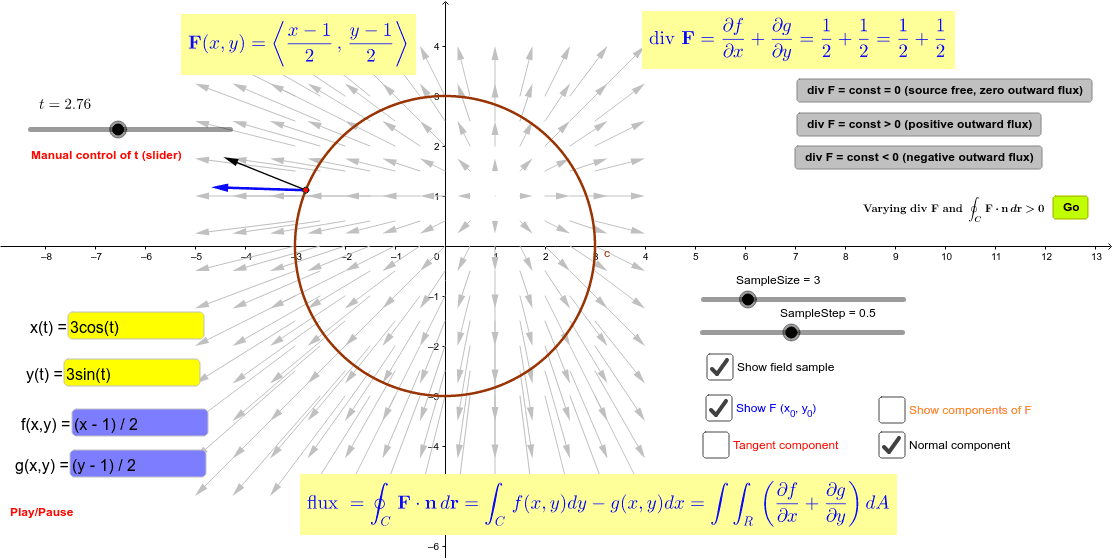
Illustration of the flux form of the Green's Theorem GeoGebra
This video explains how to determine the flux of a. Green's, stokes', and the divergence theorems 600 possible mastery points about this unit here we cover four different ways to extend the fundamental theorem of calculus to multiple dimensions. An interpretation for curl f. Web green's theorem is a vector identity which is equivalent to the curl theorem in the.
Determine the Flux of a 2D Vector Field Using Green's Theorem
Web in this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. The function curl f can be thought of as measuring the rotational tendency of. Then we state the flux form. 27k views 11 years ago line integrals. The flux of a fluid across a curve can be difficult to.
This Video Explains How To Determine The Flux Of A.
F ( x, y) = y 2 + e x, x 2 + e y. Web in this section, we examine green’s theorem, which is an extension of the fundamental theorem of calculus to two dimensions. Tangential form normal form work by f flux of f source rate around c across c for r 3. For our f f →, we have ∇ ⋅f = 0 ∇ ⋅ f → = 0.
Web Using Green's Theorem To Find The Flux.
Green's, stokes', and the divergence theorems 600 possible mastery points about this unit here we cover four different ways to extend the fundamental theorem of calculus to multiple dimensions. A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Web green’s theorem states that ∮ c f → ⋅ d r → = ∬ r curl f → d a; The discussion is given in terms of velocity fields of fluid flows (a fluid is a liquid or a gas) because they are easy to visualize.
A Circulation Form And A Flux Form, Both Of Which Require Region D In The Double Integral To Be Simply Connected.
However, green's theorem applies to any vector field, independent of any particular. The line integral in question is the work done by the vector field. Since curl f → = 0 , we can conclude that the circulation is 0 in two ways. Then we will study the line integral for flux of a field across a curve.
In This Section, We Examine Green’s Theorem, Which Is An Extension Of The Fundamental Theorem Of Calculus To Two Dimensions.
In the flux form, the integrand is f⋅n f ⋅ n. Start with the left side of green's theorem: 27k views 11 years ago line integrals. The function curl f can be thought of as measuring the rotational tendency of.