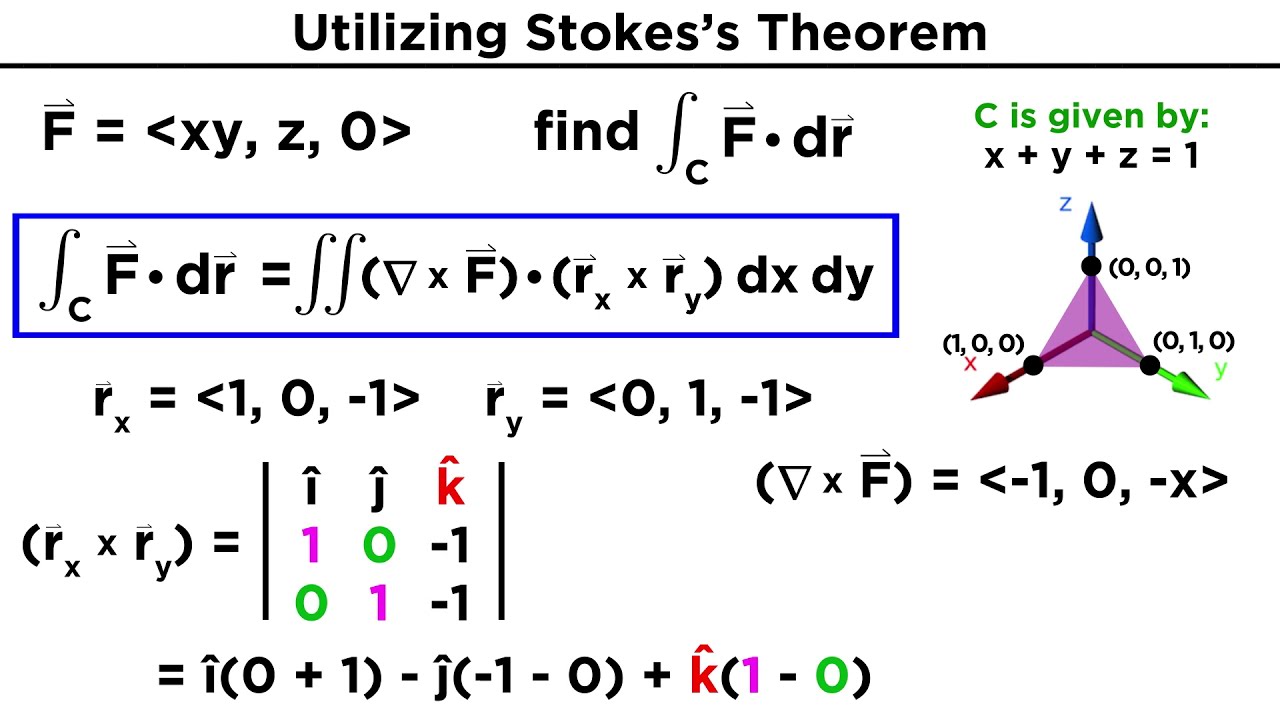
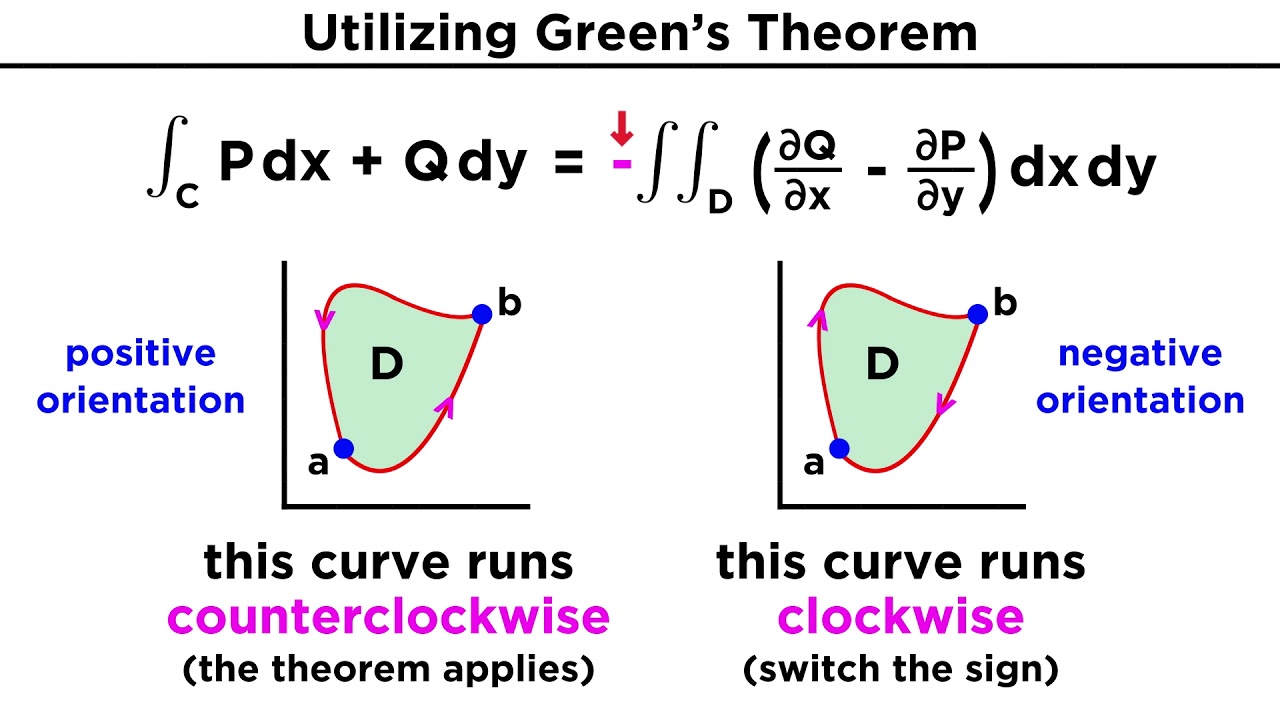
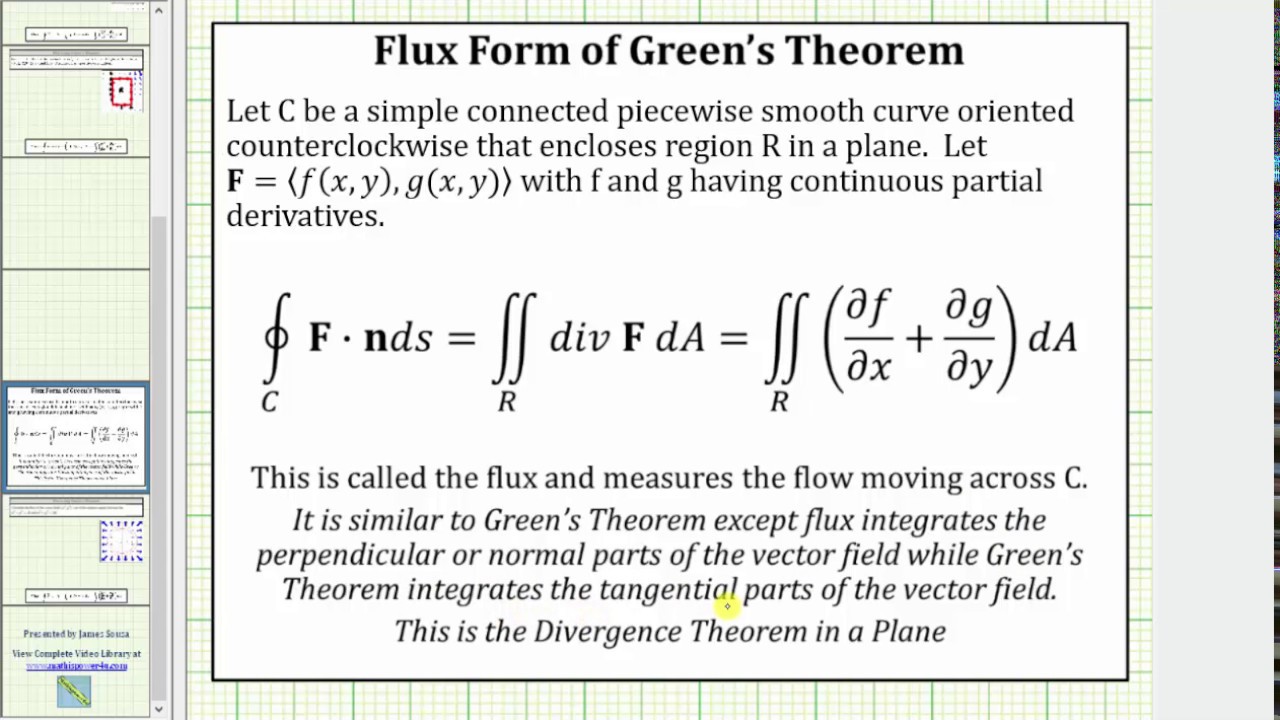
Circulation Form Of Green's Theorem
Circulation Form Of Green's Theorem - The first form of green’s theorem that we examine is the circulation form. Web theorem let c be a positively oriented, piecewise smooth, simple closed curve in a plane, and let d be the region bounded by c. If p p and q q. A circulation form and a flux form. Web green’s theorem has two forms: A circulation form and a flux form. Web green’s theorem comes in two forms: Web start circulation form of green's theorem get 3 of 4 questions to level up! In the circulation form, the integrand is f · t. What is the meaning of.
Web green’s theorem comes in two forms: Web circulation form of green's theorem math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem © 2023 khan academy terms of use. Web one thing we could do i. Web section 4.2 green's theorem (circulation form) green's theorem relates the circulation around a closed path (a global property) to the circulation density (a local. A circulation form and a flux form, both of which require region d in the double integral to be simply connected. Web green’s theorem is another higher dimensional analogue of the fundamentaltheorem of calculus: In the circulation form, the integrand is f⋅t f ⋅ t. In the flux form, the integrand is f · n. Web start circulation form of green's theorem get 3 of 4 questions to level up! Web green’s theorem comes in two forms:
A circulation form and a flux form, both of which require region d in the double integral to be simply connected. What is the meaning of. In the flux form, the integrand is f · n. Practice green's theorem (articles) learn green's theorem green's theorem examples 2d. Web section 4.2 green's theorem (circulation form) green's theorem relates the circulation around a closed path (a global property) to the circulation density (a local. It relates the line integral of a vector field around a planecurve to a double. Web this marvelous fact is called green's theorem. In the circulation form, the integrand is f · t. Web circulation form of green’s theorem. In the flux form, the integrand is f⋅n f ⋅ n.
The stokes theorem uses which of the following operation
His video is all about green's theorem, or at least the first of two green's theorem sometimes called the curl, circulation, or tangential form. Notice that green’s theorem can be used only for a two. Web green’s theorem comes in two forms: Web this marvelous fact is called green's theorem. In the flux form, the integrand is f⋅n f ⋅.
Solved The Circulation Form Of Green's Theorem Relates A
However, we will extend green’s. Web green’s theorem comes in two forms: Web start circulation form of green's theorem get 3 of 4 questions to level up! What is the meaning of. Practice green's theorem (articles) learn green's theorem green's theorem examples 2d.
Green's Theorem YouTube
If p p and q q. His video is all about green's theorem, or at least the first of two green's theorem sometimes called the curl, circulation, or tangential form. Web the circulation form of green’s theorem relates a line integral over curve c to a double integral over region d. In the circulation form, the integrand is f ·.
Green's Theorem, Circulation Form YouTube
Web green’s theorem comes in two forms: His video is all about green's theorem, or at least the first of two green's theorem sometimes called the curl, circulation, or tangential form. In the circulation form, the integrand is f · t. A circulation form and a flux form, both of which require region d in the double integral to be.
Determine the Flux of a 2D Vector Field Using Green's Theorem (Hole
A circulation form and a flux form. Math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem. Web green’s theorem has two forms: Notice that green’s theorem can be used only for a two. Web start circulation form of green's theorem get 3 of 4 questions to level up!
Curl, Circulation, and Green's Theorem // Vector Calculus YouTube
Web circulation form of green’s theorem. However, we will extend green’s. A circulation form and a flux form. Web green’s theorem has two forms: This form of the theorem relates the vector line integral over a.
Flux Form of Green's Theorem YouTube
In the circulation form, the integrand is f⋅t f ⋅ t. Practice green's theorem (articles) learn green's theorem green's theorem examples 2d. Web theorem let c be a positively oriented, piecewise smooth, simple closed curve in a plane, and let d be the region bounded by c. Web one thing we could do i. A circulation form and a flux.
Determine the Flux of a 2D Vector Field Using Green's Theorem
However, we will extend green’s. Web circulation form of green's theorem. Web section 4.2 green's theorem (circulation form) green's theorem relates the circulation around a closed path (a global property) to the circulation density (a local. This form of the theorem relates the vector line integral over a. A circulation form and a flux form.
Green's Theorem (Circulation & Flux Forms with Examples) YouTube
Web circulation form of green’s theorem. Notice that green’s theorem can be used only for a two. Web one thing we could do i. In the flux form, the integrand is f⋅n f ⋅ n. It relates the line integral of a vector field around a planecurve to a double.
multivariable calculus How are the two forms of Green's theorem are
If p p and q q. A circulation form and a flux form. Web one thing we could do i. Notice that green’s theorem can be used only for a two. Web section 4.2 green's theorem (circulation form) green's theorem relates the circulation around a closed path (a global property) to the circulation density (a local.
His Video Is All About Green's Theorem, Or At Least The First Of Two Green's Theorem Sometimes Called The Curl, Circulation, Or Tangential Form.
Web green’s theorem has two forms: A circulation form and a flux form. Web green’s theorem let c c be a positively oriented, piecewise smooth, simple, closed curve and let d d be the region enclosed by the curve. Notice that green’s theorem can be used only for a two.
It Relates The Line Integral Of A Vector Field Around A Planecurve To A Double.
If p p and q q. Web start circulation form of green's theorem get 3 of 4 questions to level up! Web circulation form of green’s theorem. Practice green's theorem (articles) learn green's theorem green's theorem examples 2d.
In The Flux Form, The Integrand Is F · N.
The first form of green’s theorem that we examine is the circulation form. This form of the theorem relates the vector line integral over a. Web one thing we could do i. Web green’s theorem is another higher dimensional analogue of the fundamentaltheorem of calculus:
Web Green’s Theorem Comes In Two Forms:
If l and m are functions of (x, y) defined on an. Math > multivariable calculus > green's, stokes', and the divergence theorems > green's theorem. Web this marvelous fact is called green's theorem. In the circulation form, the integrand is f⋅t f ⋅ t.